
Compound interest is a powerful concept that allows your investments to grow exponentially over time. In this article, we will explore the compound interest formula calculator and its role in helping you understand and maximize your investment growth. We will delve into the intricacies of compound interest, explain how to calculate it manually, compare it to simple interest, discuss factors that affect compound interest calculations, and provide real-life applications and tips for maximizing your returns.
Compound Interest Explained
Compound interest refers to the interest earned not only on the initial principal amount but also on the accumulated interest from previous periods. Unlike simple interest, which only takes into account the principal amount, compound interest allows your investment to grow at an accelerated rate. This compounding effect can significantly boost your returns over time.
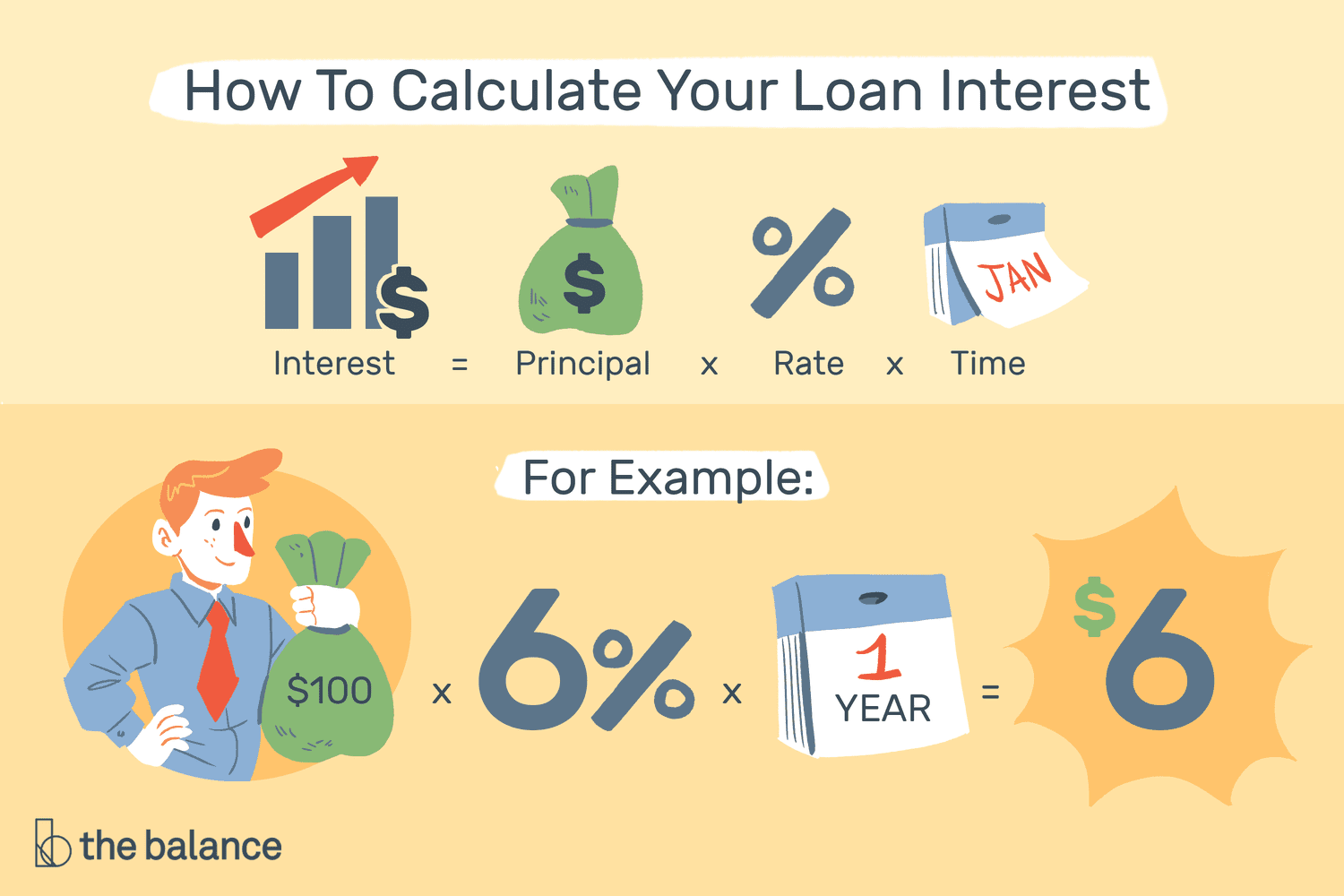
To better understand compound interest, let’s consider an example. Suppose you invest $1,000 in a savings account with an annual interest rate of 5%. At the end of the first year, you would earn $50 in interest (5% of $1,000). In the second year, however, you wouldn’t just earn another $50; instead, you would earn interest on the increased principal amount of $1,050. This compounding effect continues as long as your investment remains active, resulting in exponential growth.
How to Calculate Compound Interest
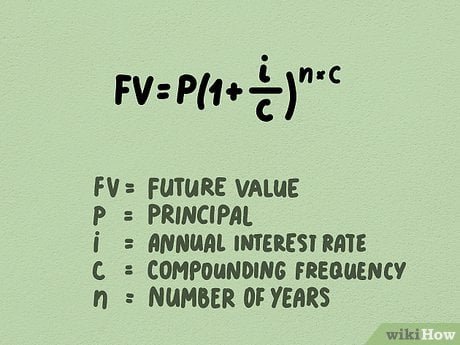
Calculating compound interest may seem complex, but with the compound interest formula, it becomes straightforward. The compound interest formula is as follows:
A = P(1 + r/n)^(nt)
where:
- A represents the future value of the investment, including both the principal and compounded interest.
- P is the principal amount (the initial investment).
- r is the annual interest rate (expressed as a decimal).
- n denotes the number of times that interest is compounded per year.
- t represents the number of years the money is invested for.
Let’s illustrate this formula using our previous example. We have an initial investment of $1,000 (P), an annual interest rate of 5% (r = 0.05), compounded annually (n = 1), and a time period of 3 years (t).
By substituting these values into the formula, we can calculate the future value of the investment (A):
A = 1000(1 + 0.05/1)^(1*3) A = 1000(1 + 0.05)^3 A = 1000(1.05)^3 A ≈ 1000(1.157625) A ≈ $1,157.63
The future value of the investment would be approximately $1,157.63 after three years, considering the compound interest.
Understanding the Compound Interest Formula
To gain a deeper understanding of the compound interest formula, let’s break it down further:
- The term (1 + r/n) represents the growth factor associated with each compounding period. It adds the interest rate (r) divided by the number of compounding periods per year (n) to 1.
- The exponent (nt) accounts for the total number of compounding periods over the investment’s duration (t). It multiplies the number of compounding periods per year (n) by the number of years (t).
By raising the growth factor to the power of the total number of compounding periods, we capture the compounding effect and arrive at the future value of the investment (A).
Understanding these components helps you grasp how each variable contributes to the overall growth of your investment. By manipulating the values in the compound interest formula, you can assess different scenarios and make informed decisions about your investments.
Benefits of Using a Compound Interest Calculator
Calculating compound interest manually can be tedious and time-consuming, especially when dealing with complex investment scenarios. This is where a compound interest calculator becomes invaluable. By utilizing a compound interest calculator, you can easily determine the future value of your investments based on different parameters.
One of the significant benefits of using a compound interest calculator is its ability to provide quick and accurate results. With just a few inputs, such as the principal amount, interest rate, compounding frequency, and investment duration, the calculator can generate precise calculations of the future value of your investment.
Additionally, a compound interest calculator allows you to explore various investment scenarios by adjusting the input values. You can experiment with different interest rates, compounding frequencies, and investment durations to assess how they impact your investment growth. This flexibility empowers you to make informed decisions and strategize your investment plans effectively.
Steps to Calculate Compound Interest Manually
While using a compound interest calculator is convenient, understanding the manual calculation process is essential for developing a thorough grasp of compound interest. Here are the steps to calculate compound interest manually:
- Identify the principal amount (P), the annual interest rate (r), the number of compounding periods per year (nContinued:
- Determine the investment duration in years (t).
- Divide the annual interest rate by the number of compounding periods per year to obtain the periodic interest rate. Let’s denote this as i = r/n.
- Multiply the number of compounding periods per year by the investment duration to obtain the total compounding periods (nt).
- Add 1 to the periodic interest rate (i) and raise it to the power of the total compounding periods (nt). This gives us (1 + i)^nt.
- Multiply the principal amount (P) by the result from step 5 to calculate the future value of the investment (A).
Let’s use our previous example to demonstrate the manual calculation process. We have P = $1,000, r = 5%, n = 1, and t = 3.
Step 1: Identify the variables:
- P = $1,000 (principal amount)
- r = 5% (annual interest rate)
- n = 1 (compounding periods per year)
- t = 3 (investment duration)
Step 2: Calculate the periodic interest rate (i):
- i = r/n = 0.05/1 = 0.05
Step 3: Determine the total compounding periods (nt):
- nt = n t = 1 3 = 3
Step 4: Calculate (1 + i)^nt:
- (1 + i)^nt = (1 + 0.05)^3 = 1.05^3 = 1.157625
Step 5: Multiply the principal amount (P) by (1 + i)^nt:
- A = P (1 + i)^nt = $1,000 1.157625 = $1,157.63
By following these steps, we manually calculate the future value of the investment to be approximately $1,157.63, which matches the result obtained using the compound interest formula.
Compound Interest vs. Simple Interest
Compound interest and simple interest are two methods used to calculate the growth of an investment. While simple interest only applies to the initial principal amount, compound interest takes into account the accumulated interest from previous periods, resulting in exponential growth.
To illustrate the difference between compound interest and simple interest, let’s consider an example. Suppose you have $1,000 invested at an annual interest rate of 5% for three years.
Using simple interest, the calculation would be straightforward:
- Simple interest = P r t = $1,000 0.05 3 = $150
Hence, after three years, the investment would accumulate $150 in interest, resulting in a total value of $1,150 ($1,000 + $150).
However, when using compound interest, the calculation becomes more complex, but the growth potential is significantly higher. As we previously calculated, the future value of the investment after three years at a 5% annual interest rate with compounding would be approximately $1,157.63.
The key difference lies in the compounding effect, where the interest earned in each period is added to the principal, leading to higher returns over time. Compound interest allows your investment to grow exponentially, making it a more favorable option for long-term investments.
Factors Affecting Compound Interest Calculations
Several factors can affect compound interest calculations. Understanding these factors helps you make informed decisions and optimize your investment growth. Here are some key factors to consider:
- Principal Amount (P): The initial investment amount plays a crucial role in determining the future value of your investment. The higher the principal amount, the greater the growth potential.
- Annual Interest Rate (r): The interest rate directly impacts the rate at which your investment grows. A higher interest rate leads to faster accumulation of compounded interest.
- Compounding Frequency (n): The number of compounding periods per year affects the frequency at which interest is added to the principal. More frequent compounding results in more significant growth over time.
- Investment Duration (t): The length of time your money remains invested influences the overall growth potential. Longer investment durations allow for more compounding periods, resulting in higher returns.
By considering these factors and adjusting your investment strategy accordingly, you can maximize the power of compound interest and optimize your investment growth.
Using a Compound Interest Formula for Investment Planning
A compound interest formula calculator serves as a valuable tool for investment planning. By utilizing this calculator, you can forecast the future value of your investments based on different scenarios and make well-informed decisions. Here’s how you can use a compound interest formula for investment planning:
- Determine your financial goal: Define the purpose of your investment and set a specific financial goal. For example, you might aim to save for retirement, a down payment on a house, or a child’s education fund.
- IdentifyContinued:
- Identify your investment timeframe: Determine the duration for which you are willing to keep your money invested. The longer the investment timeframe, the greater the potential for compound interest to work its magic.
- Research investment options: Explore different investment opportunities such as stocks, bonds, mutual funds, or real estate. Assess their historical performance, risk levels, and expected returns.
- Estimate the potential returns: Use a compound interest formula calculator to estimate the future value of your investment based on various parameters like principal amount, interest rate, compounding frequency, and investment duration. Adjust these variables to explore different scenarios and understand the potential growth of your investment.
- Evaluate risk and return trade-offs: Consider the level of risk associated with different investment options and determine an appropriate balance between risk and expected returns. Higher-risk investments may offer higher returns but also come with increased volatility.
- Monitor and review: Regularly monitor the performance of your investments and review your financial goals. Adjust your investment strategy if necessary to maximize your returns and align with changing circumstances.
By using a compound interest formula calculator in your investment planning, you can set realistic financial goals, make informed investment decisions, and optimize your wealth accumulation over time.
Common Mistakes to Avoid When Calculating Compound Interest
While calculating compound interest, it’s important to be aware of common mistakes that can lead to inaccurate results. By avoiding these pitfalls, you can ensure precise calculations and make sound financial decisions. Here are some common mistakes to watch out for:
- Incorrectly applying the formula: Ensure that you use the correct formula for compound interest and substitute the values accurately. Double-check your calculations to prevent errors.
- Neglecting to account for compounding periods: Be mindful of the number of compounding periods per year when using the formula. Using the wrong value for “n” can result in incorrect calculations.
- Failing to convert interest rates: If the annual interest rate is given as a percentage, remember to convert it to a decimal before using it in the formula. For example, 5% should be expressed as 0.05.
- Using inconsistent units of time: Ensure that the time period matches the compounding frequency. If the interest rate is annual, make sure the investment duration is in years and vice versa for other compounding frequencies.
- Ignoring additional contributions or withdrawals: If you are making regular contributions or withdrawals during the investment period, the compound interest formula alone may not provide an accurate representation. Consider adjusting the calculations to include these factors if necessary.
By avoiding these mistakes and maintaining accuracy in your compound interest calculations, you can rely on the results to guide your investment decisions effectively.
Real-Life Applications of Compound Interest
Compound interest has numerous real-life applications that demonstrate its impact on various financial scenarios. Understanding these applications can help you appreciate the power of compound interest and make informed financial choices. Here are some examples:
- Savings accounts: When you deposit money into a savings account, compound interest allows your savings to grow over time. The more frequently interest compounds, the faster your savings will accumulate.
- Retirement planning: Compound interest plays a crucial role in retirement planning. By starting early and consistently contributing to a retirement account, you can benefit from the compounding effect over several decades, allowing your savings to grow significantly by the time you retire.
- Investment portfolios: Investors utilize compound interest when building diversified portfolios. The reinvestment of dividends and capital gains can lead to exponential growth, enhancing the overall returns generated by the portfolio.
- Student loans and mortgages: On the flip side, compound interest can also work against you when borrowing money. With student loans or mortgages, the accumulated interest adds to the principal, resulting in higher repayment amounts over time.
- Debt repayment strategies: On the positive side, you can leverage compound interest to accelerate debt repayment. By making extra principal payments on loans with higher interest rates, you can reduce the total interest paid over the loan term.
These real-life applications demonstrate the significance of compound interest in various financial contexts. Whether it’s growing your savings, planning for retirement, or managing debt, understanding and utilizing compound interest can help you achieve your financial goals more effectively.
Tips for Maximizing Compound Interest
To make the most of compound interest and optimize your investment growth, consider the following tips:
- Start early: The power of compound interest is amplified with time. Start investing as early as possible to benefit from the compounding effect over a longer period.
- Be consistent: Regularly contribute to your investments to maximize the growth potential. Consistency allows you to take advantage of compounding and ensure steady progress towards your financial goals.
- Increase contribution amounts: As your income grows, consider increasing your investment contributions. Higher contributions lead to larger principal amounts, resulting in more substantial compound interest earnings.
- Choose investments wisely: Select investment options that offer competitive interest rates and strong growth potential. Diversify your portfolio toContinued:
- Choose investments wisely: Select investment options that offer competitive interest rates and strong growth potential. Diversify your portfolio to spread the risk and maximize potential returns.
- Reinvest dividends and interest: If you receive dividends or interest from your investments, consider reinvesting them rather than withdrawing them. This allows for continuous compounding and accelerates the growth of your investment.
- Take advantage of tax-advantaged accounts: Explore tax-advantaged investment accounts such as IRAs or 401(k)s, which offer tax benefits that can enhance your investment growth.
- Review and adjust your strategy: Regularly review your investment performance and goals. Make adjustments as needed to ensure your investment strategy aligns with your changing financial circumstances.
- Minimize fees and expenses: Keep an eye on investment fees and expenses, as they can eat into your returns over time. Choose low-cost investment options whenever possible.
- Stay informed: Stay updated on market trends, economic news, and investment opportunities. This knowledge can help you make informed decisions and capitalize on favorable investment conditions.

By implementing these tips, you can optimize the power of compound interest and maximize your investment growth over time.
In conclusion, understanding compound interest and using a compound interest formula calculator is essential for comprehending the growth potential of your investments. By leveraging the compounding effect, you can accelerate your wealth accumulation and work towards achieving your financial goals. Remember to consider factors like principal amount, interest rate, compounding frequency, and investment duration when using the compound interest formula calculator. With proper planning, disciplined investing, and a long-term perspective, you can harness the power of compound interest to secure a financially prosperous future.






